Page 106 - The Indian Optician Digital Edition May-Junel 2022

To convert the sum back to its sphero- and the sum of the lenses is -0.73 / +3.46 x 45.
cylindrical form, the resultant cylinder, CR, is first
Two further examples are given below
calculated from C = √(C + C 45 2 ). to confirm the application of the method
2
R
0
Either the plus or minus root can be taken to of astigmatic decomposition to compound
give the plus or minus cylinder transposition as obliquely combined cylinders.
required.
EXAMPLE (II) FROM PART ONE
The axis of the resultant cylinder, θ , is given
R
by tan θR = (C – C ) /C Find the sphero-cylinder equivalent to the
R 0 45 following pair of crossed cylinders: +1.00 DC x 30
If the axis resulting from this expression turns / -4.00 DC x 60.
out to be negative, simply add 180 to it to obtain Lens 1: (+1.00 DC x 30)
the axis direction in Standard Notation.
MRE = S + C/2 = 0 + 1/2 = +0.50
The resultant sphere, S , is given by:
R C = C cos 2θ = 1 cos 60 = +0.50
0
total MRE - C /2 C = C sin 2θ = 1 sin 60 = +0.8660
R
45
As an example, the sum of the two lenses: Lens 2: (-4.00 DC x 60)
+2.00/+2.00 x 30 and -1.00/-2.00 x 150 yields the
following: MRE = S + C/2 = 0 + -4/2 = -2.00
Lens 1: C = C cos 2θ = -4 cos 60 = +2.00
0
C = C sin 2θ = -4 sin 60 = -3.464
MRE = S + C/2 = 2 + 2/2 = +3 45
C = C cos 2θ = 2 cos 60 = +1
0
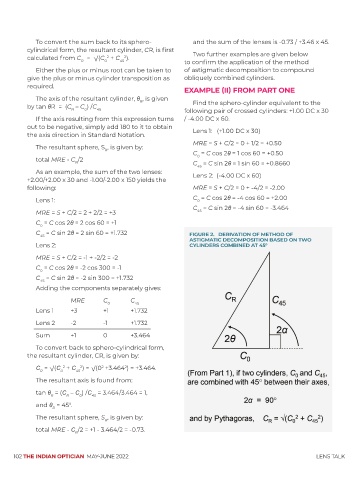
C = C sin 2θ = 2 sin 60 = +1.732 FIGURE 2. DERIVATION OF METHOD OF
45
ASTIGMATIC DECOMPOSITION BASED ON TWO
Lens 2: CYLINDERS COMBINED AT 45°
MRE = S + C/2 = -1 + -2/2 = -2
C = C cos 2θ = -2 cos 300 = -1
0
C = C sin 2θ = -2 sin 300 = +1.732
45
Adding the components separately gives:

MRE C C 45
0
Lens 1 +3 +1 +1.732

Lens 2 -2 -1 +1.732
Sum +1 0 +3.464

To convert back to sphero-cylindrical form,
the resultant cylinder, CR, is given by:

2
2
C = √(C + C 45 2 ) = √(0 +3.464 ) = +3.464.
2
0
R
The resultant axis is found from:
tan θ = (C – C ) /C = 3.464/3.464 = 1,
R R 0 45
and θ = 45°.
R
The resultant sphere, S , is given by:
R
total MRE - C /2 = +1 - 3.464/2 = -0.73.
R

102 THE INDIAN OPTICIAN MAY-JUNE 2022 LENS TALK