Page 117 - The Indian Optician Digital Edition March-April 2021

A similar expression can be employed method of doing this by employing a polynomial
to determine the cylindrical power obtained surface of the form
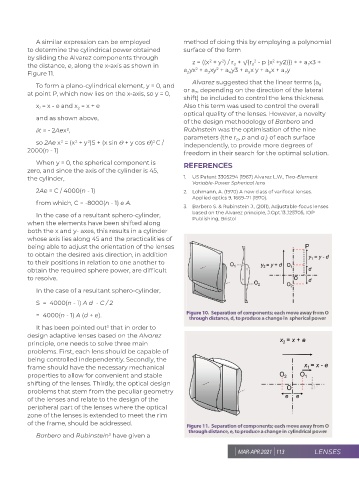
by sliding the Alvarez components through 2 2 2 2
0
0
1
the distance, e, along the x-axis as shown in z = ((x + y ) / r + √{r - p (x +y2)}) + + a x3 +
2
2
3
4
6
7
Figure 11. a yx + a xy + a y3 + a x y + a x + a y
5
2
Alvarez suggested that the linear terms (a
To form a plano-cylindrical element, y = 0, and 6
or a , depending on the direction of the lateral
at point P, which now lies on the x-axis, so y = 0, 7
shift) be included to control the lens thickness.
x = x - e and x = x + e Also this term was used to control the overall
1
2
optical quality of the lenses. However, a novelty
and as shown above,
of the design methodology of Barbero and
୿t = - 2Aex , Rubinstein was the optimisation of the nine
2
parameters (the r , p and a ) of each surface
0
i
2
2
2
2
so 2Ae x = (x + y )S + (x sin ת + y cos ת) C /
independently, to provide more degrees of
2000(n - 1)
freedom in their search for the optimal solution.
When y = 0, the spherical component is REFERENCEES
E
zero, and since the axis of the cylinder is 45,
the cylinder, 1. US Patent 3305294 (1967) Alvarez L.W., Two-Element
Variable-Power Spherical lens
2Ae = C / 4000(n - 1) 2. Lohmann, A. (1970) A new class of varifocal lenses.
Applied optics 9, 1669–71 (1970).
from which, C = -8000(n - 1) e A.
3. Barbero S. & Rubinstein J., (2011), Adjustable-focus lenses
based on the Alvarez principle, J.Opt.13.,125705, IOP
In the case of a resultant sphero-cylinder,
Publishing, Bristol
when the elements have been shifted along
both the x and y- axes, this results in a cylinder
whose axis lies along 45 and the practicalities of
being able to adjust the orientation of the lenses
to obtain the desired axis direction, in addition
to their positions in relation to one another to
obtain the required sphere power, are difficult
to resolve.
In the case of a resultant sphero-cylinder,
S = 4000(n - 1) A d - C / 2
y
a
m
i
= 4000(n - 1) A (d + e). Figure 10. Separation of compoonents; each move aaway froom O
w
t through distancee, d, to produce a change in spherical power
c
r
It has been pointed out that in order to
3
design adaptive lenses based on the Alvarez
principle, one needs to solve three main
problems. First, each lens should be capable of
being controlled independently. Secondly, the
frame should have the necessary mechanical
properties to allow for convenient and stable
shifting of the lenses. Thirdly, the optical design
problems that stem from the peculiar geometry
of the lenses and relate to the design of the
peripheral part of the lenses where the optical
zone of the lenses is extended to meet the rim
of the frame, should be addressed.
m
i
a
Figure 11. Separation of compoonents; each move aawayy froom O
t through distance, e, to producee a change in ccylindrrical powwer
e
3
Barbero and Rubinstein have given a
| MAR-APR 2021 | 113 LENSES
Mar-Apr 2021 SK.indd 61 26-04-2021 13:32