Page 116 - The Indian Optician Digital Edition March-April 2021

and the thickness of the second element at P is

2
2
t = t - Ay (x + ⅓y ).
2
2
P2
C
Substituting y - d for y and y + d for y , leads to
1
2
2
2
t = t + A(y - d)(x + ⅓(y - d) )
C
P1
2
and t = t - A(y + d)(x2 + ⅓(y + d) ).
P2
C
Adding these to obtain the combined
thickness variation at P, gives:
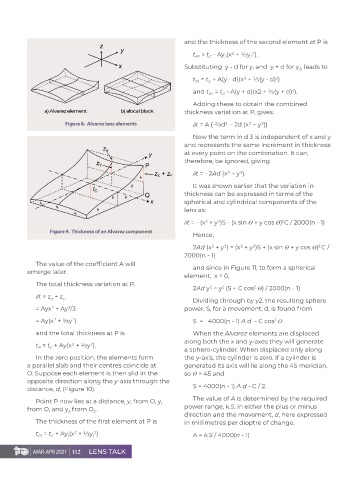
Figgure 8. Alvareez lens elements ୿t = A {-⅔d - 2d (x + y )}
2
2
3
n
Now the term in d 3 is independent of x and y
and represents the same increment in thickness
at every point on the combination. It can,
therefore, be ignored, giving
2
2
୿t = - 2Ad (x + y ).
It was shown earlier that the variation in
thickness can be expressed in terms of the
spherical and cylindrical components of the
lens as:
2
2
୿t = - (x + y )S - (x sin ת + y cos ת) C / 2000(n - 1)
2
Figure 9. Thickness of aan Alvarez componeent
m
Hence,
2
2Ad (x + y ) = (x + y )S + (x sin ת + y cos ת) C /
2
2
2
2
2000(n - 1)
The value of the coefficient A will
and since in Figure 11, to form a spherical
emerge later.
element, x = 0,
The total thickness variation at P,
2
2Ad y = y (S + C cos ת) / 2000(n - 1)
2
2
୿t = z + z
X Y Dividing through by y2, the resulting sphere
3
= Ayx + Ay /3 power, S, for a movement, d, is found from
2
2
2
= Ay(x + ⅓y ) S = 4000(n - 1) A d - C cos ת
2
and the total thickness at P is When the Alvarez elements are displaced
along both the x and y-axes they will generate
2
2
t = t + Ay(x + ⅓y ).
C
P
a sphero-cylinder. When displaced only along
In the zero position, the elements form the y-axis, the cylinder is zero. If a cylinder is
a parallel slab and their centres coincide at generated its axis will lie along the 45 meridian,
O. Suppose each element is then slid in the so ת = 45 and
opposite direction along the y-axis through the
S = 4000(n - 1) A d - C / 2.
distance, d, (Figure 10).
The value of A is determined by the required
Point P now lies at a distance, y, from O, y 1
from O and y from O . power range, k.S, in either the plus or minus
1
2
2
direction and the movement, d, here expressed
The thickness of the first element at P is in millimetres per dioptre of change.
2
t = t + Ay (x + ⅓y ) A = k.S / 4000(n - 1)
2
1
1
C
P1
| MAR-APR 2021 | 112 LENS TALK
Mar-Apr 2021 SK.indd 60 26-04-2021 13:32