Page 113 - The Indian Optician Digital Edition July-August 2021

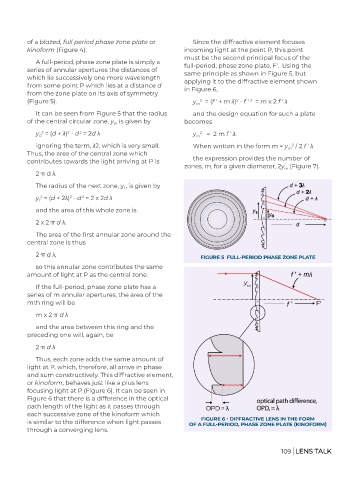
of a blazed, full period phase zone plate or Since the diffractive element focuses
kinoform (Figure 4). incoming light at the point P, this point
must be the second principal focus of the
A full-period, phase zone plate is simply a full-period, phase zone plate, F'. Using the
series of annular apertures the distances of same principle as shown in Figure 5, but
which lie successively one more wavelength applying it to the diffractive element shown
from some point P which lies at a distance d in Figure 6,
from the zone plate on its axis of symmetry
(Figure 5). y = (f ' + m λ) - f ' = m x 2 f ' λ
2
2
2
m
It can be seen from Figure 5 that the radius and the design equation for such a plate
of the central circular zone, y , is given by becomes
0
2
2
2
y = (d + λ) - d = 2d λ y = 2 m f ' λ.
2
0
m
ignoring the term, λ2, which is very small. When written in the form m = y / 2 f ' λ
2
m
Thus, the area of the central zone which
contributes towards the light arriving at P is the expression provides the number of
zones, m, for a given diameter, 2y (Figure 7).
m
2 π d λ.
The radius of the next zone, y , is given by
1
2
2
2
y = (d + 2λ) - d = 2 x 2d λ
1
and the area of this whole zone is
2 x 2 π d λ.
The area of the first annular zone around the
central zone is thus
2 π d λ, FIGURE 5 FULL-PERIOD PHASE ZONE PLATE
so this annular zone contributes the same
amount of light at P as the central zone.
If the full-period, phase zone plate has a
series of m annular apertures, the area of the
mth ring will be
m x 2 π d λ

and the area between this ring and the
preceding one will, again, be

2 π d λ
Thus, each zone adds the same amount of
light at P, which, therefore, all arrive in phase
and sum constructively. This diffractive element,
or kinoform, behaves just like a plus lens
focusing light at P (Figure 6). It can be seen in
Figure 6 that there is a difference in the optical
path length of the light as it passes through
each successive zone of the kinoform which
FIGURE 6 - DIFFRACTIVE LENS IN THE FORM
is similar to the difference when light passes OF A FULL-PERIOD, PHASE ZONE PLATE (KINOFORM)
through a converging lens.

109 | LENS TALK